Truncated Octahedron

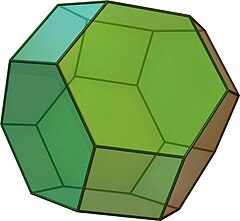
also known as a mecon.

A truncated octahedron is one of a few shapes known to tile R3, and it is a member of a category of structures, called "PermutaHedra?", that tile RN. The next simpler category member, the hexagon, tiles R2 in permutationally equivalent ways.

A truncated octahedron has 14 flats. If you stick 14 more truncated octahedrons onto those flats, they form a larger structure, itself shaped like a truncated octahedron. Further, to travel from the exact center of the innermost truncated octahedron straight into any outer truncated octahedron you can only cross a flat. Crossing a corner puts you inside a flat. Contrast cubes, where traveling over a corner puts you into a diagonal cube.

exam question: Assemble 15 truncated octahedrons into a ball, with no air between them. Shrink down very small, and stand on an outer flat of one ot the truncated octahedrons. To reach another truncated octahedron you can walk down this one, and step over onto the next one. You must step over an edge (the border of an inner flat). If you step over a corner, you will land on an edge.

How many different truncated octahedrons can you step onto from any original truncated octahedron, crossing one edge? Is this number the same for every truncated octahedron on the surface?

next question: Take fifteen clusters of 15 packed truncated octahedrons each. Build a super cluster. Now what are the numbers of truncated octahedrons you can step onto?

See also: GeneralizedBalancedTernary, which uses TO's as a data structure.

TruncatedOctahedrons are fascinating objects. The intersection of a cube and a regular octahedron, if you connect the centers of their 8 hexes you get two intersecting tetrahedra. If you connect these up you can make a phenomenally strong and beautiful 3-level living space out of them - diagram to follow. Nice flat floors and ceilings but not too many vertical walls, I'm afraid ...