Klein Bottle

A surface with no "inside" or "outside". In three dimensions, a KleinBottle must intersect itself, but not in four or more dimensions.

From http://www-groups.dcs.st-and.ac.uk/~history/Mathematicians/Klein.html "We should also mention the Klein bottle, a one-sided closed surface named after Klein. A Klein bottle cannot be constructed in Euclidean space. It is best pictured as a cylinder looped back through itself to join with its other end. However this is not a continuous surface in 3-space as the surface cannot go through itself without a discontinuity. It is possible to construct a Klein bottle in non-Euclidean space..."

CliffordStoll makes and sells KleinBottles (and Klein steins). See http://www.kleinbottle.com/.

From http://www.greencheese.us/ZeekLandWeekNine (which see to understand why Pigleg is tied to the table and Poo is hiding a running chain saw behind his back...)

---

Take two disks, and deform each one just a little until they are bowls. Disks only have one edge, so each bowl has only one edge, so now glue the edge of one to the edge of the other, and what you get is a sphere (well, distorted somewhat). Since each disk has two sides the sphere also has two sides, only now it's an inside and an outside.

Got that?

OK, now consider the MoebiusStrip. Just as a disk has only one edge, so the MoebiusStrip has only one edge. We can imagine taking two MoebiusStrips and gluing the edge of one to the edge of the other. Now we have a KleinBottle.

It's a bit hard to do in reality because the twistiness gets in the way a bit, but we can imagine doing it.

---

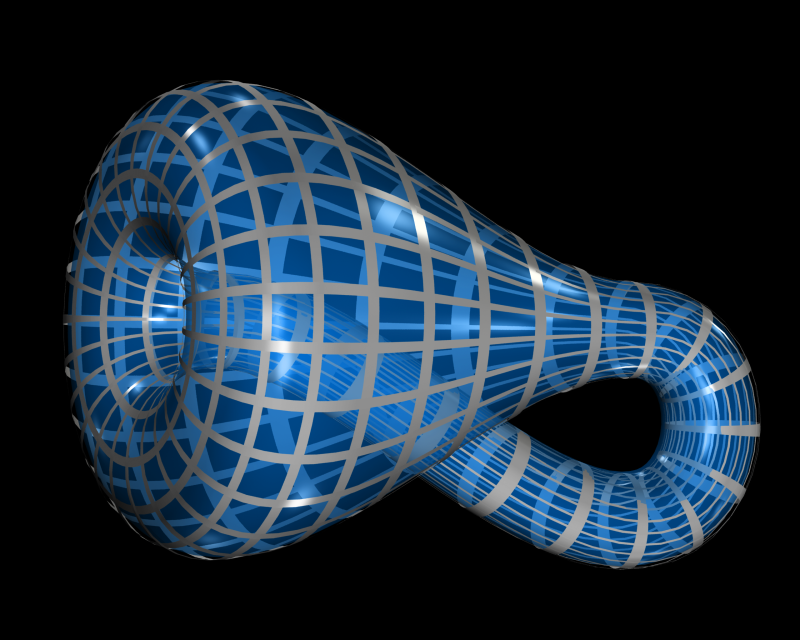
The Banchoff Klein Bottle

• http://alem3d.obidos.org/en/struik/kbottle/
• http://www.google.com/search?q=%22Klein+Bottle%22
• http://www.andrewlipson.com/kleinbottle.htm
• http://mathworld.wolfram.com/KleinBottle.html

---

Not quite the elegance of the MoebiusStrip, though. There is a definite finiteness to it. The water goes in the tube and around and into the bottle and then it has nowhere to go but back through the tube. The MoebiusStrip has the wonderful quality that you can start drawing a line on one side and keep going in the same direction until you eventually arrive back at the place you started.

Surely the analogy should be of something more viscous, like oil, flowing down the inner surface of the tube, coating the inside of the bottle then up the outside of the tube to return to the start (ignoring the barrier, which is only there in 3d space). The point being that we've only gone up one dimension from a line to a surface, not to a volume.

I guess I'm having a hard time picturing it in four dimensions!

Most people have trouble picturing things in four dimensions. I'm working on that.

Try this. Colour the bottle in the above picture with a colour ranging from red to blue. If the tube is narrow, make it really red, if the tube is wide make it really blue. Now think of the colour as being your extra dimension. Two things are in the same 4-D place if they have the same 3-D co-ordinates and have the same colour. Where the narrow part of the tube apparently meets the wide part of the tube they are in fact in different 4-D places because they have different colours.

Of course, you're not being helped by the fact that the "pretty" 3-D embedding shown above does not show the full symmetry.

---

Consider the poem in The SpaceChildsMotherGoose?, by Frederick Winsor and Marian Parry, ISBN 1-930-900-07-4 :

  Three jolly sailors from Blaydon-on-Tyne
  They went to sea in a bottle by Klein.
  Since the sea was entirely inside the hull
  The scenery seen was exceedingly dull.

---

Or this limerick:

  A mathematician named Klein
  thought the Möbius strip was divine.
He said, "If you glue
the edges of two,
  you'll get a weird bottle like mine."

---

CategoryMath